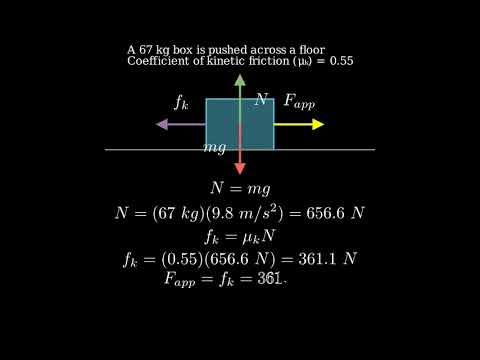
You push a 67-kg box across a floor where the coefficient of kinetic friction is μk = 0.55. The force you exert is horizontal. (a) How much power is needed to push the box at a speed of 0.50 m/s? (b) How much work do you do if you push the box for 35 s? .
**Power and Work Calculation for Pushing a Box**

- Given:
- Mass of the box (m) = 67 kg
- Coefficient of kinetic friction (μk) = 0.55
- Speed (v) = 0.50 m/s
- Time (t) = 35 s
- Gravitational acceleration (g) = 9.8 m/s²

1. **Force due to friction (F_friction):**
- The force of friction is given by: \( F_{\text{friction}} = \mu_k \cdot F_{\text{normal}} \)
- Where the normal force, \( F_{\text{normal}} \), is equal to the gravitational force: \( F_{\text{normal}} = m \cdot g \)
- \( F_{\text{normal}} = 67 \, \text{kg} \cdot 9.8 \, \text{m/s}^2 = 656.6 \, \text{N} \)
- \( F_{\text{friction}} = 0.55 \cdot 656.6 \, \text{N} \)
- \( F_{\text{friction}} \approx 360.1 \, \text{N} \)

2. **(a) Power Calculation:**
- Power is given by: \( P = F_{\text{friction}} \cdot v \)
- \( P = 360.1 \, \text{N} \cdot 0.50 \, \text{m/s} \)
- \( P \approx 180.05 \, \text{W} \)

- **Result (a):**
The power needed to push the box at a speed of 0.50 m/s is approximately **180.05 W**.

3. **(b) Work Calculation:**
- Work is given by: \( W = F_{\text{friction}} \cdot d \)
- First, calculate the distance traveled: \( d = v \cdot t \)
- \( d = 0.50 \, \text{m/s} \cdot 35 \, \text{s} = 17.5 \, \text{m} \)
- Now, calculate the work:
\( W = 360.1 \, \text{N} \cdot 17.5 \, \text{m} \)
- \( W \approx 6301.75 \, \text{J} \)

- **Result (b):**
The work done in 35 s is approximately **6301.75 J**.